Momentum
Momentum is a measure of how easily something can be brought to rest. A rocket moving through the sound barrier is going to have a much larger momentum than your nan tottering down the road to the post office.
Momentum, mass and velocity
Momentum can be calculated using the following equation:
This tells us that object with a higher mass and travelling at a higher velocity will have a larger momentum. Since velocity is a vector quantity, momentum is also a vector quantity (it has direction).
Providing that no external forces are acting on an object, its momentum will be conserved during a collision or explosion. This means that the total momentum before a collision will be the same after the crash as before.
Worked example: conservation of momentum
A lorry travelling along the motorway loses control and crashes into a car which was stationary on the lay-by, causing both vehicles to slide away together. The lorry has a mass of 10,000 kg and was moving at a speed of 8 m/s before the collision. The car has a mass of 1,300 kg and was stationary. What is their velocity after the crash?
- Work out the momentum before the collision using the equation momentum = mass x velocity
- Momentum of the lorry = 10,000 x 8 = 80,000 kg m/s
- Momentum of the car = 1,300 x 0 = 0 kg m/s
- Total momentum before the collision = 80,000 + 0 = 80,000 kg m/s
- We know that momentum is conserved so the momentum after the collision is also 80,000 kg m/s
- We then rearrange the equation to determine velocity: velocity = momentum / mass
- Velocity = 80,000 / (10,000 + 1,300) = 7.1 m/s2
Newton’s second law of motion
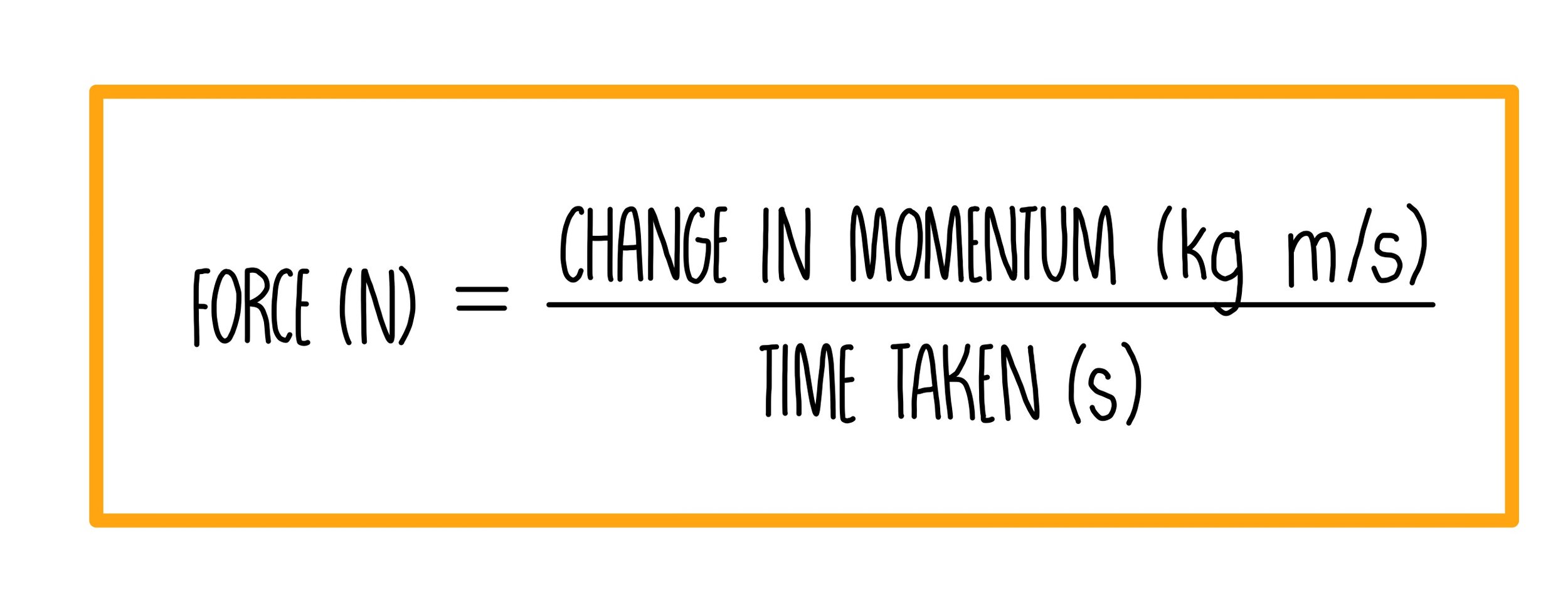
Isaac Newton showed that the rate of change of momentum is proportional to the size of the force acting on the object. Force can be calculated using the equation:
To change the momentum of an object you can apply a small force over a long time, or a larger force over a shorter time. For example, if I am driving a car and want to come to a stop, I can either press really hard on the break over a short time or press very gently over a longer amount of a time.
Worked example: force and momentum
During a tennis match Serena Williams hits a 50 g tennis ball at a velocity of 70 m/s in 5 seconds. With what force did she hit the ball?
We first need to work out the momentum of the ball using the equation momentum = mass x velocity. Remember that mass is always in kg.
Momentum = 0.05 kg x 70 m/s = 3.5 kg m/s
We can then use the equation force = change in momentum / time
Force = 3.5 / 5 = 0.7 N
Crumple zones, air bags and seat belts all increase the time taken for the change of momentum during a car crash. When time increases, the force on the passengers is reduced.
Car safety features
During a car crash, the car decelerates and comes to a stop very quickly. This means that the time taken to change momentum is very short. Using the force = change in momentum / time equation, we can see that if the value for time is very small then the force will be very large. It is dangerous for passengers to experience a large force so it is important to increase the time taken to bring the car to rest. Seat belts, air bags and crumple zones all increase the time taken for the car (or passenger) to come to a stop and so reduce the force on the passengers, reducing possible injuries.
Newton’s third law of motion
Newton’s third law states that when two objects interact, they exert equal and opposite forces on each other. For example, when pushing a trolley the trolley pushes the person backwards as the person pushes the trolley forwards. These contact forces are equal in size and opposite in direction.
Moments
A moment is the turning effect of a force. For example, when I apply a force by pushing my bike pedals, this creates a moment by turning the bike wheels. Moments act about a point (called the pivot) in a clockwise or anticlockwise direction. Levers are used to increase the turning effect of a force by increasing the distance between the force applied and the point at which the object turns.
Worked example: calculating moments
A force of 60 N is used to turn a bolt using a spanner. The perpendicular distance between the spanner and the pivot is 30 cm. Calculate the moment.
Use the equation: moment = force x perpendicular distance from the pivot. Remember to convert distance into meters.
Moment = 60 x 0.3 = 18 Nm
If an object is balanced, this means that the turning effect acting in the clockwise direction is equal to the turning effect in the anticlockwise direction. Let’s say if two people of equal weight are sat at equal distances from the pivot on a see-saw, the clockwise and anticlockwise moments will be balanced and the sea-saw will stay still.
Worked example: clockwise and anticlockwise moments
Two children sit on a see-saw made from a plank 8 m long pivoted in the middle. One child sits 1.5 m from the middle of the plank and weighs 250 N. If the other child weighs 400 N, where must she sit so that the sea-saw is balanced?
- We know that if the sea-saw is balanced, the clockwise moment must be equal to the anticlockwise moment.
- Sum of the clockwise moment = force x distance from pivot
- Moment = 250 N x 1.5 m = 375 Nm
- This means that the anticlockwise moment = 375 Nm.
- Distance from pivot = moment / force
- Distance = 375 Nm / 400 N = 0.94 m